Parabolas with Vertices at the Origin
Learning Objectives
- Identify and label the focus, directrix, and endpoints of the focal diameter of a parabola
- Write the equation of a parabola given a focus and directrix
 Parabola
Parabola Key features of the parabola
Key features of the parabola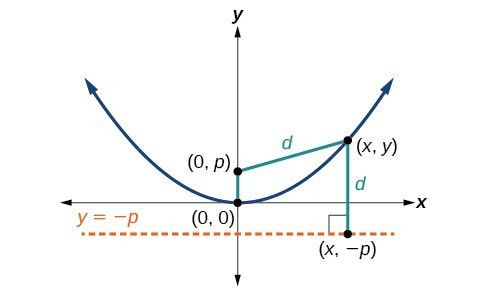 Let be a point on the parabola with vertex , focus , and directrix as shown in Figure 4. The distance from point to point on the directrix is the difference of the y-values: . The distance from the focus to the point is also equal to and can be expressed using the distance formula.
Let be a point on the parabola with vertex , focus , and directrix as shown in Figure 4. The distance from point to point on the directrix is the difference of the y-values: . The distance from the focus to the point is also equal to and can be expressed using the distance formula.
Set the two expressions for equal to each other and solve for to derive the equation of the parabola. We do this because the distance from to equals the distance from to . We then square both sides of the equation, expand the squared terms, and simplify by combining like terms.
The equations of parabolas with vertex are when the x-axis is the axis of symmetry and when the y-axis is the axis of symmetry. These standard forms are given below, along with their general graphs and key features.
A General Note: Standard Forms of Parabolas with Vertex (0, 0)
The table below summarizes the standard features of parabolas with a vertex at the origin.| Axis of Symmetry | Equation | Focus | Directrix | Endpoints of Focal Diameter |
| x-axis | ||||
| y-axis |
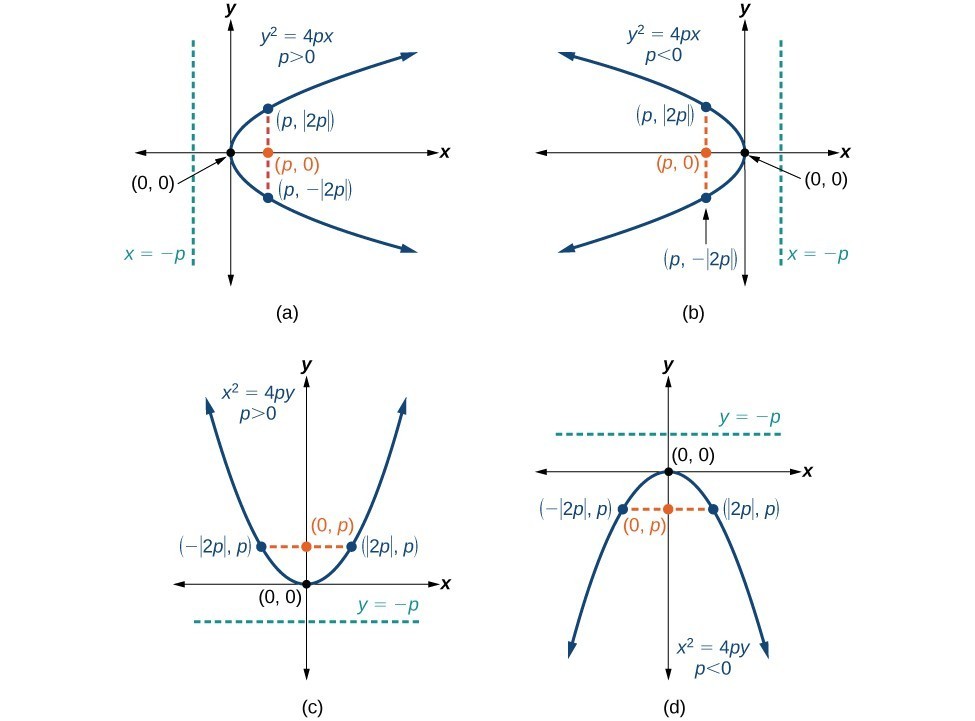 (a) When and the axis of symmetry is the x-axis, the parabola opens right. (b) When and the axis of symmetry is the x-axis, the parabola opens left. (c) When and the axis of symmetry is the y-axis, the parabola opens up. (d) When and the axis of symmetry is the y-axis, the parabola opens down.
(a) When and the axis of symmetry is the x-axis, the parabola opens right. (b) When and the axis of symmetry is the x-axis, the parabola opens left. (c) When and the axis of symmetry is the y-axis, the parabola opens up. (d) When and the axis of symmetry is the y-axis, the parabola opens down.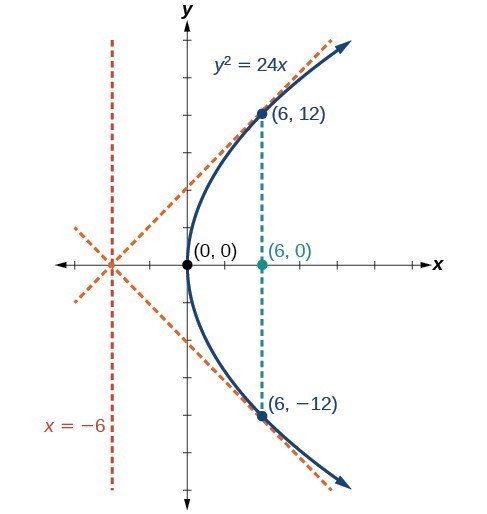
How To: Given a standard form equation for a parabola centered at (0, 0), sketch the graph.
- Determine which of the standard forms applies to the given equation: or .
- Use the standard form identified in Step 1 to determine the axis of symmetry, focus, equation of the directrix, and endpoints of the focal diameter.
- If the equation is in the form , then
- the axis of symmetry is the x-axis,
- set equal to the coefficient of x in the given equation to solve for . If , the parabola opens right. If , the parabola opens left.
- use to find the coordinates of the focus,
- use to find the equation of the directrix,
- use to find the endpoints of the focal diameter, . Alternately, substitute into the original equation.
- If the equation is in the form , then
- the axis of symmetry is the y-axis,
- set equal to the coefficient of y in the given equation to solve for . If , the parabola opens up. If , the parabola opens down.
- use to find the coordinates of the focus,
- use to find equation of the directrix,
- use to find the endpoints of the focal diameter,
- If the equation is in the form , then
- Plot the focus, directrix, and focal diameter, and draw a smooth curve to form the parabola.
Example: Graphing a Parabola with Vertex (0, 0) and the x-axis as the Axis of Symmetry
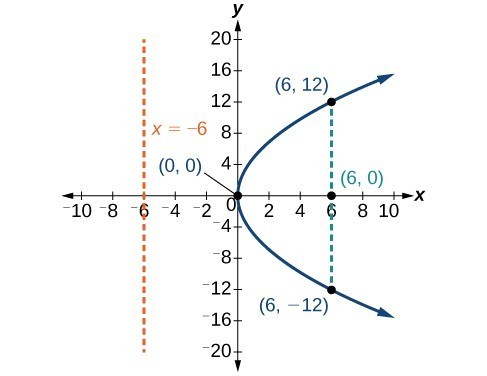
Graph . Identify and label the focus, directrix, and endpoints of the focal diameter.Answer: The standard form that applies to the given equation is . Thus, the axis of symmetry is the x-axis. It follows that:
- , so . Since , the parabola opens right the coordinates of the focus are
- the equation of the directrix is
- the endpoints of the focal diameter have the same x-coordinate at the focus. To find the endpoints, substitute into the original equation:
Try It
Graph . Identify and label the focus, directrix, and endpoints of the focal diameter.Answer:
Focus: ; Directrix: ; Endpoints of the latus rectum:

